Share
Gavin deposited money into his savings account that is compounded annually at an interest rate of 9%. Gavin thought the equivalent quarterly
Question
Gavin deposited money into his savings account that is compounded annually at an interest rate of 9%. Gavin thought the equivalent quarterly interest rate would be 2.25%. Is Gavin correct? If he is, explain why. If he is not correct, state what the equivalent quarterly interest rate is and show how you got your answer.
in progress
0
Mathematics
3 years
2021-08-22T17:00:04+00:00
2021-08-22T17:00:04+00:00 1 Answers
27 views
0

Answers ( )
Answer:
The equivalent rate is of 0.093 = 9.3% a year. Gavin is wrong, because he applied the wrong formula, its not just dividing the interest rate r by the number of compoundings n, it is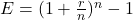
Step-by-step explanation:
Equivalent interest rate:
The equivalent interest rate for an amount compounded n times during a year is given by:
In which r is the interest rate and n is the number of compoundings during an year.
Compounded annually at an interest rate of 9%
This means that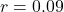
Compounded quarterly:
This means that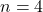
Equivalent rate:
The equivalent rate is of 0.093 = 9.3% a year. Gavin is wrong, because he applied the wrong formula, its not just dividing the interest rate r by the number of compoundings n, it is