a golf ball is launched straight up with an initial velocity of 15.68m/s. Calculate the balls displacement and velocity every 0.4 sec into t

Question

a golf ball is launched straight up with an initial velocity of 15.68m/s. Calculate the balls displacement and velocity every 0.4 sec into the ball’s flight. How long will it take the ball in the air?​

in progress 0
Huyền Thanh 3 years 2021-07-25T20:20:53+00:00 2 Answers 20 views 0

Answers ( )

  1. niczorrrr
    0
    2021-07-25T20:22:18+00:00
    Reply

    Answer:

    calculated velocities:

    (t,v(t))

    (0.4,11.756)

    (0.8,7.832)

    (1.2,3.908)

    (1.6,-0.016)

    calculated displacements:

    (t,\Delta x)

    (0.4, 5.487)

    (0.8,9.4048)

    (1.2, 11.7528)

    (1.6, 12.5312)

    the ball spends 3.2 seconds in the air

    Explanation:

    Assuming the ball is launched on earth with standard gravity and that the effects of drag is neglected.

    assume that ‘up’ is positive direciton since that is direction of travel

    ‘down’ is negative direction

    formula for velocity is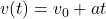 v(t)=v_0+at

    where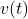 v(t) is the velocity at time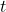 t

    v_0 is the initial velocity at time t=0

    a is the acceleration in the direction of the velocity

    velocity is in m/s and accelration is in m/s^2 and t is in seconds

    for displacement: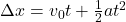 \Delta x=v_0 t+\frac{1}{2}at^2

    Known stuffs:

    in our case, we know we are on earth so,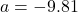 a=-9.81m/s^2. the negative sign indicates that the direction is oposite the velocity since the ball travels up but gravity pulls down

    the initial velocity is given as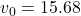 v_0=15.68m/s

    therefore, our equation for velocity is

    v(x)=15.68-9.81t

    now we just need to plot it every 0.4 seconds

    this is easy subsituteion. here’s the first:

    v(0.4)=15.68-9.81(0.4)=11.756m/s

    if we keep plotting this, we get the following table

    (t,v(t))

    (0.4,11.756)

    (0.8,7.832)

    (1.2,3.908)

    (1.6,-0.016) the velocity is negative meaning that the ball is now going ‘down’ and therefor the ball has just passed the peak of it’s upward travel

    so since it took about 1.6 seconds to get here, it will spend about twice this time in the air or about 3.2 seconds

    to get an exact answer, solve for the time when velocity is 0, ie when the ball has reached its peak and stopped moving

    set v(t)=0 and solve for t

    0=15.68-9.81t\rightarrow 15.68=9.81t\rightarrow t=1.598s

    so it takes 1.598 seconds to get to peak, therefore it spends twice this time in the air or 3.197 seconds in the air. it’s close enough to 3.2 seconds

    the ball spends 3.2 seconds in the air

    displacement:

    \Delta x=v_0t+\frac{1}{2}at^2

    \Delta x=15.68t+\frac{1}{2}(-9.81)t^2

    some values are

    (t,\Delta x)

    (0.4, 5.487)

    (0.8,9.4048)

    (1.2, 11.7528)

    (1.6, 12.5312)

  2. Verity
    0
    2021-07-25T20:22:21+00:00
    Reply

    Explanation:

    Given:

    v₀ = 15.68 m/s

    a = -9.8 m/s²

    t = 0.4n s, n = 0, 1, 2, etc.

    Find: Δy and v

    To find displacement, use the equation:

    Δy = v₀ t + ½ at²

    To find velocity, use the equation:

    v = at + v₀

    \left\begin{array}{ccc}t&y&v\\0&0&15.68\\0.4&5.49&11.76\\0.8&9.41&7.84\\1.2&11.76&3.92\\1.6&12.54&0\\2.0&11.76&-3.92\\2.4&9.41&-7.84\\2.8&5.49&-11.76\\3.2&0&-15.68\end{array}\right

    The ball lands after 3.2 seconds.

Leave an answer

Browse

Giải phương trình 1 ẩn: x + 2 - 2(x + 1) = -x . Hỏi x = ? ( )