A quantity of gas with an initial volume of 5 cubic feet and a pressure of 1700 pounds per square foot expands to a volume of 9 cubic feet.

Question

A quantity of gas with an initial volume of 5 cubic feet and a pressure of 1700 pounds per square foot expands to a volume of 9 cubic feet. Find the work done by the gas for the given volume and pressure. Round your answer to two decimal places. Assume the temperature of the gas in this process remain constant.

in progress 0
RI SƠ 3 years 2021-07-16T19:39:56+00:00 1 Answers 8 views 0

Answers ( )

  1. diemthu
    0
    2021-07-16T19:40:57+00:00
    Reply

    Answer:

    Work done by the gas for the given volume and pressure = 4996.18 pounds foot

    Explanation:

    Given

    Pressure applied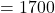 = 1700 pounds per square foot

    Initial Volume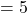 = 5 cubic feet

    Final Volume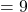 = 9 cubic feet

    As we know pressure is inversely proportional to V

    P = \frac{k}{V}

    where k is the proportionality constant

    V is the volume and

    P is the pressure

    Work done

    \int\limits^{V_2}_{V_1} {P} \, dV

     \int\limits^{V_2}_{V_1} {\frac{k}{V} } \, dV\\= \int\limits^{V_2}_{V_1} {\frac{1700 * 5}{V} } \, dV\\= 8500* \int\limits^{V_2}_{V_1} {\frac{1}{V} } \, dV

    Integrating the above equation, we get-

    8500 ln \frac{V_2}{V_1} \\8500 * 2.303 * \frac{9}{5} \\= 4996.18

    Work done by the gas for the given volume and pressure = 4996.18 pounds foot

Leave an answer

Browse

Giải phương trình 1 ẩn: x + 2 - 2(x + 1) = -x . Hỏi x = ? ( )