Which explicit formula is equivalent to a1 = 1, an= 4an-1? A. an = 1(4)^n-1 B. an = 4(4)^n-1 C. an = 4(1)^n–1

Question

Which explicit formula is equivalent to a1 = 1, an=
4an-1?
A. an = 1(4)^n-1
B. an = 4(4)^n-1
C. an = 4(1)^n–1
D. an = 1 + (n – 1)4

in progress 0
Ladonna 3 years 2021-09-05T14:47:13+00:00 1 Answers 29 views 0

Answers ( )

  1. Euphemia
    0
    2021-09-05T14:48:50+00:00
    Reply

    Answer:

    A. an = 1(4)^n-1

    Step-by-step explanation:

    Geometric sequence:

    In a geometric sequence, the quotient between consecutive terms is always the same, called common ratio, and the sequence is given by:

    a_n = a_1(q)^{n-1}

    In which a_1 is the first term and q is the common ratio.

    We are given the following sequence:

    a_1 = 1

    a_n = 4a_{n-1}

    So

    a_2 = 4a_1 = 4

    a_3 = 4a_2 = 16

    a_4 = 4a_3 = 64

    That is, the quotient between consecutive terms is 4, so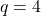 q = 4.

    The first term is already given,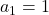 a_1 = 1. So

    a_n = a_1(q)^{n-1}

    a_n = 1(4)^{n-1}

    And thus, the correct answer is given by option A.

Leave an answer

Browse

Giải phương trình 1 ẩn: x + 2 - 2(x + 1) = -x . Hỏi x = ? ( )